The Power of the Bernoulli Principle
Did you know that the laws of physics can explain how airplanes fly, how water flows in a river, and even how you breathe? It’s all thanks to the remarkable power of the Bernoulli Principle and the fascinating field of fluid dynamics. In this article, we will delve into the science behind the Bernoulli Principle and discover how it governs the behavior of fluids in motion.
Prepare to be amazed by the invisible forces that shape the world around us and learn how this powerful principle impacts our daily lives. Get ready for a journey into the mesmerizing realm of fluid mechanics!
Understanding Fluid Dynamics
Fluid dynamics means how fluids, both liquids and gases, behave when they are in motion. It is a branch of physics that explores the forces and pressures exerted by fluids as they flow, and it has applications in a wide range of fields, from engineering to medicine.
Bernoulli Principle, named after the Swiss scientist Daniel Bernoulli who first formulated it in the 18th century. This principle states that as the speed of a fluid increases, its pressure decreases. In other words, when a fluid is flowing rapidly, it exerts less pressure than when it is flowing slowly.
The Bernoulli Equation and Its Components
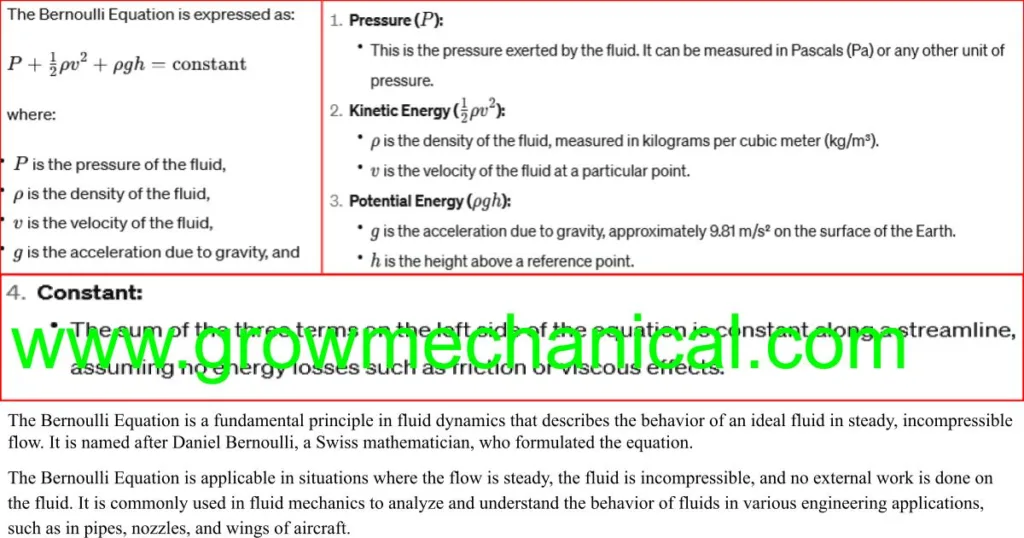
We need to explore the Bernoulli equation, which mathematically describes the relationship between fluid speed, pressure, and height. The equation is as follows:
The general form of the Bernoulli Equation is: P+1/2ρv2+ρgh=constant
where:
- is the pressure of the fluid,
- is the density of the fluid,
- is the velocity of the fluid,
- is the acceleration due to gravity,
- is the height of the fluid above a reference point.
The equation states that the sum of the pressure energy, kinetic energy, and potential energy per unit volume of the fluid is constant along a streamline (a path traced by a fluid particle in motion).
Now, let’s break down the components of the Bernoulli Equation:
- Pressure ():
- Represents the internal pressure of the fluid.
- It can include static pressure, dynamic pressure, and any other form of pressure energy.
- Kinetic Energy (1/2ρv2):
- Describes the energy associated with the motion of the fluid.
- is the density of the fluid.
- is the velocity of the fluid.
- Potential Energy ):
- Relates to the gravitational potential energy of the fluid.
- ρ is the density of the fluid.
- is the acceleration due to gravity.
- is the height of the fluid above a reference point.
- Constant:
- The sum of these three energy components is constant along a streamline.
This equation shows that the sum of the pressure, kinetic energy per unit volume, and potential energy per unit volume of a fluid remains constant along a streamline. In simpler terms, it means that as the speed of the fluid increases, either the pressure or the height must decrease to maintain the constant value.
Applications of the Bernoulli Principle in Everyday Life
The Bernoulli Principle has a wide range of applications in our everyday lives, many of which we may not even be aware of. Let’s explore a few examples to see how this principle influences various aspects of our daily experiences.
Airplanes:
The Bernoulli Principle is crucial in the flight of airplanes. As an airplane moves through the air, the shape of its wings causes the air to move faster over the top surface compared to the bottom surface. According to the Bernoulli Principle, faster-moving air creates lower pressure, resulting in higher pressure underneath the wings, providing lift and allowing the airplane to fly.
Water Fountains:
Water fountains operate on the Bernoulli Principle. As water flows through a narrow nozzle, its velocity increases, resulting in lower pressure according to Bernoulli’s equation. This lower pressure causes water to be drawn up from the reservoir, creating the characteristic upward stream of water seen in fountains.
Wind Turbines:
Wind turbines utilize the Bernoulli Principle to generate electricity. As wind flows over the blades of a turbine, it accelerates, creating an area of lower pressure. This pressure difference between the front and back of the blades causes them to rotate, driving the turbine’s generator and producing electricity.
The Role of the Bernoulli Principle in Plumbing Systems
In plumbing systems, the Bernoulli Principle plays a significant role in regulating fluid flow. For instance, when water travels through narrow pipes or fittings, its velocity increases, leading to a decrease in pressure according to Bernoulli’s Principle. This pressure drop enables efficient movement of water through the pipes and allows for the operation of various fixtures such as faucets, showers, and toilets.
Understanding the Bernoulli Principle
The Bernoulli Principle states that as the speed of a fluid increases, its pressure decreases, and vice versa, provided the fluid’s flow is steady and there is no external work being done on it. This principle is fundamental in fluid dynamics and is applied in various real-world scenarios, from explaining the lift of airplane wings to the operation of water fountains and plumbing systems. Understanding Bernoulli’s Principle helps engineers and scientists design efficient systems and devices across numerous fields, from aviation to hydraulics.
Famous Experiments Related to the Bernoulli Principle
One of the most famous experiments related to the Bernoulli Principle is the Venturi Effect, named after Italian physicist Giovanni Battista Venturi. In this experiment, Venturi observed that as the velocity of a fluid increases, its pressure decreases. This phenomenon is crucial in understanding how fluids behave in various situations, from airflow over an airplane wing to the flow of water through pipes.
Challenges and Limitations of the Bernoulli Principle
While the Bernoulli Principle provides valuable insights into fluid behavior, it has its limitations and challenges. One challenge is its applicability to real-world scenarios where factors like viscosity, turbulence, and compressibility come into play. Additionally, accurately applying the Bernoulli Principle requires understanding and accounting for all contributing factors, which can be complex in practical engineering applications.
The Bernoulli Principle in Sports
The Bernoulli Principle plays a significant role in sports, particularly in activities like aerodynamics in cycling and racing cars. Athletes and engineers leverage this principle to design equipment and vehicles that maximize speed and efficiency. For example, streamlined helmets and vehicle shapes are engineered to minimize air resistance and take advantage of the Bernoulli effect to gain a competitive edge.
The Bernoulli Principle in Engineering and Design
Engineers and designers frequently utilize the Bernoulli Principle in various fields such as aviation, HVAC systems, and fluid dynamics. Understanding how changes in fluid velocity affect pressure allows engineers to optimize designs for efficiency and performance. Applications range from aircraft wing design to optimizing the airflow in ventilation systems to ensure efficient heating and cooling.
Experimental Demonstrations of the Bernoulli Principle
Numerous experiments demonstrate the Bernoulli Principle’s effects, such as the classic demonstration involving airflow over a curved surface. This experiment illustrates how the faster-moving air above a curved surface creates lower pressure, resulting in lift. Other demonstrations include the Bernoulli bag experiment, where blowing air between two sheets of paper causes them to come together due to reduced pressure.
Calculating Fluid Flow Using Bernoulli’s Equation
Bernoulli’s equation provides a mathematical framework for calculating fluid flow by accounting for changes in pressure, velocity, and elevation along a streamline. Engineers and scientists use this equation to analyze and predict fluid behavior in various systems, from water distribution networks to hydraulic machinery. By applying Bernoulli’s equation, they can optimize system performance and troubleshoot potential issues.
Conclusion: The Limitless Potential of the Bernoulli Principle in Various Industries
The Bernoulli Principle’s versatility and applicability across numerous industries highlight its immense potential. From revolutionizing aircraft design to enhancing sports equipment performance, this principle continues to shape technological advancements. Despite its challenges and limitations, ongoing research and innovation ensure that the Bernoulli Principle remains a cornerstone of modern engineering and design, offering solutions to complex fluid dynamics problem.
